Auxetic Dihedral Escher Tessellations
CAD 2020 (Presented on SPM 2020, Best Paper Award, 1st Place)
1Shandong University 2University of Konstanz

The dual shapes 𝑺𝟏 and 𝑺𝟐 are deformed into 𝑷𝟏 and 𝑷𝟐 which can be rotated and deployed without obstructing each other.
Abstract
The auxetic structure demonstrates an unconventional deployable mechanism, expanding in transverse directions while being stretched longitudinally (exhibiting a negative Poisson's ratio).
This characteristic offers advantages in diverse fields such as structural engineering, flexible electronics, and medicine.
The rotating (semi-)rigid structure, as a typical auxetic structure, has been introduced into the field of computer-aided design because of its well-defined motion patterns.
These structures find application as deployable structures in various endeavors aiming to approximate and rapidly fabricate doubly-curved surfaces, thereby mitigating the challenges associated with their production and transportation.
Nevertheless, prior designs relying on basic geometric elements primarily concentrate on exploring the inherent nature of the structure and often lack aesthetic appeal.
To address this limitation, we propose a novel design and generation method inspired by dihedral Escher tessellations.
By introducing a new metric function, we achieve efficient evaluation of shape deployability as well as filtering of tessellations, followed by a two-step deformation and edge-deployability optimization process to ensure compliance with deployability constraints while preserving semantic meanings.
Furthermore, we optimize the shape through physical simulation to guarantee deployability in actual manufacturing and control Poisson's ratio to a certain extent.
Our method yields structures that are both semantically meaningful and aesthetically pleasing, showcasing promising potential for auxetic applications.
Pipeline
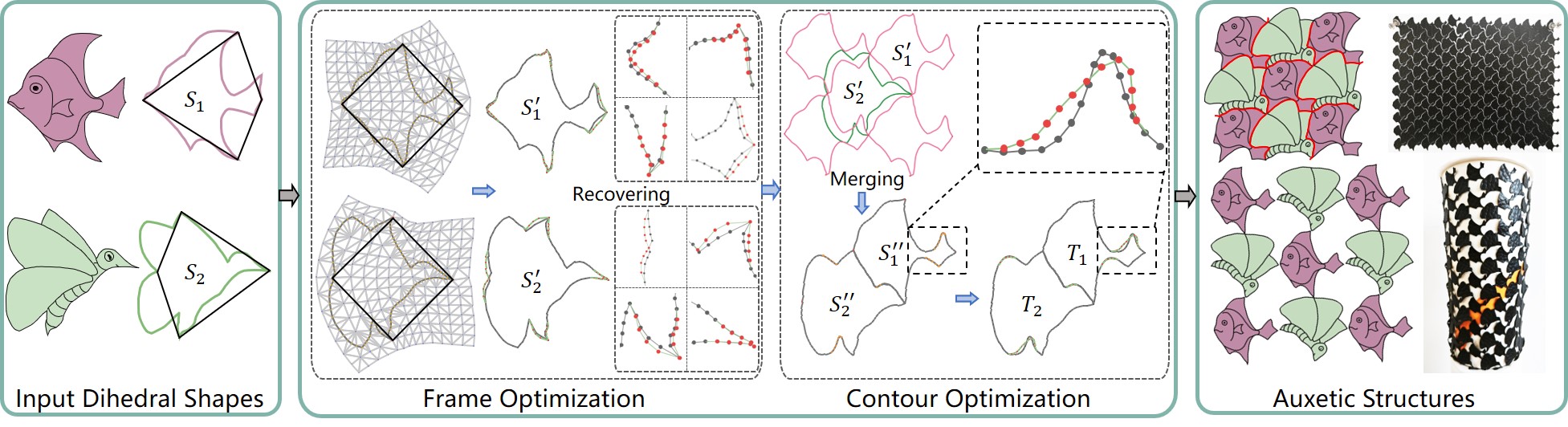
Starting with the dual shapes of a dihedral tessellation, we first deform them separately using a two-step ARAP-based deformation method to transform their anchor frames into a regular geometric unit (here a rhombus). Then, we merge the two shapes and perform a deployability optimisation based on a deployability metric function to obtain the final deployable dihedral Escher tessellationVarious dual-shape tiling patterns generated by our system.
Auxetic Dihedral Tessellation
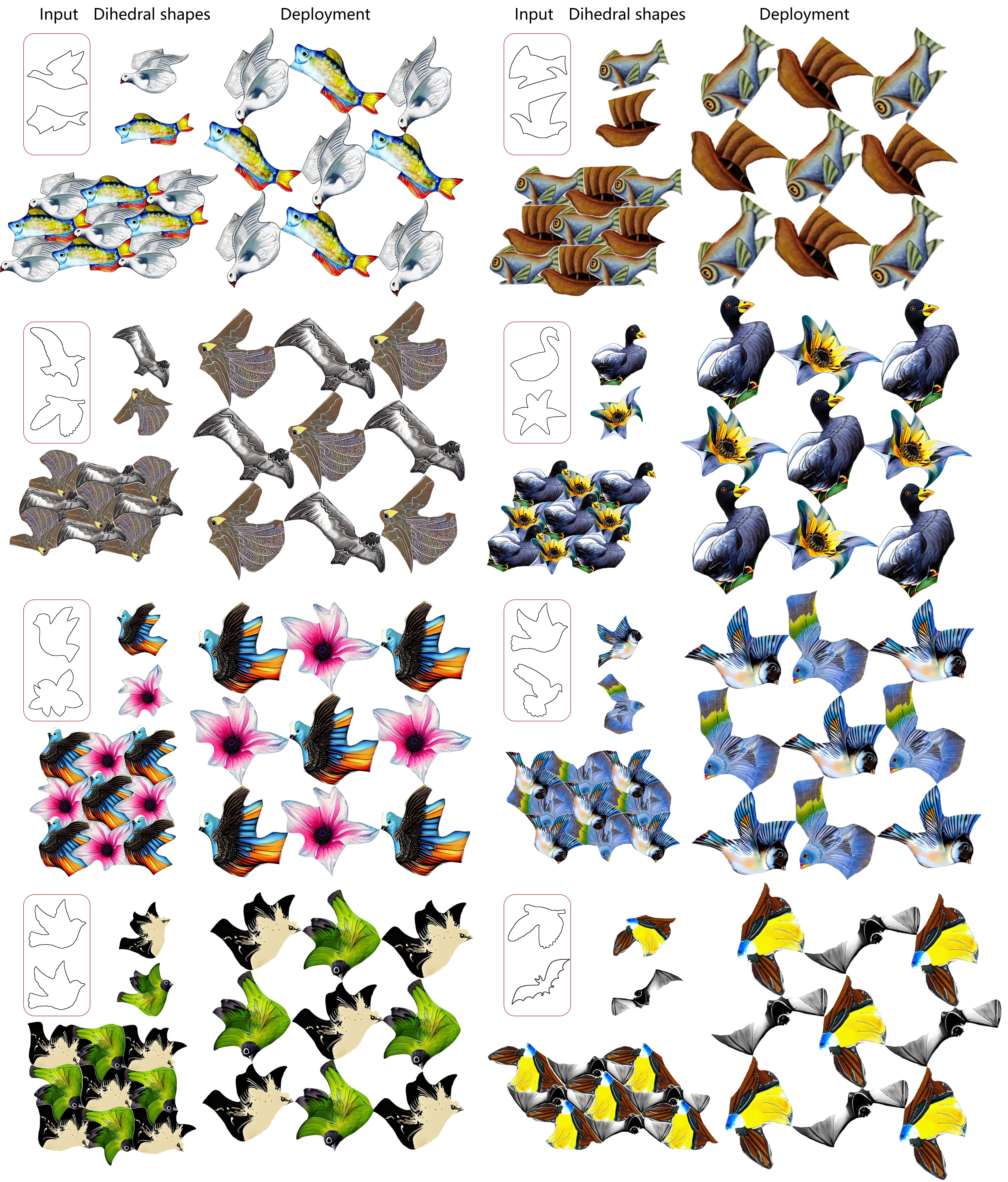
Auxetic dihedral Escher tessellations. The top row is obtained through deployability optimization on Escher’s hand-drawn artwork, while the remaining three rows are based on newly generated dual-shape tessellations.
Fabricable models

Auxetic leather tape fabricated through laser cutting
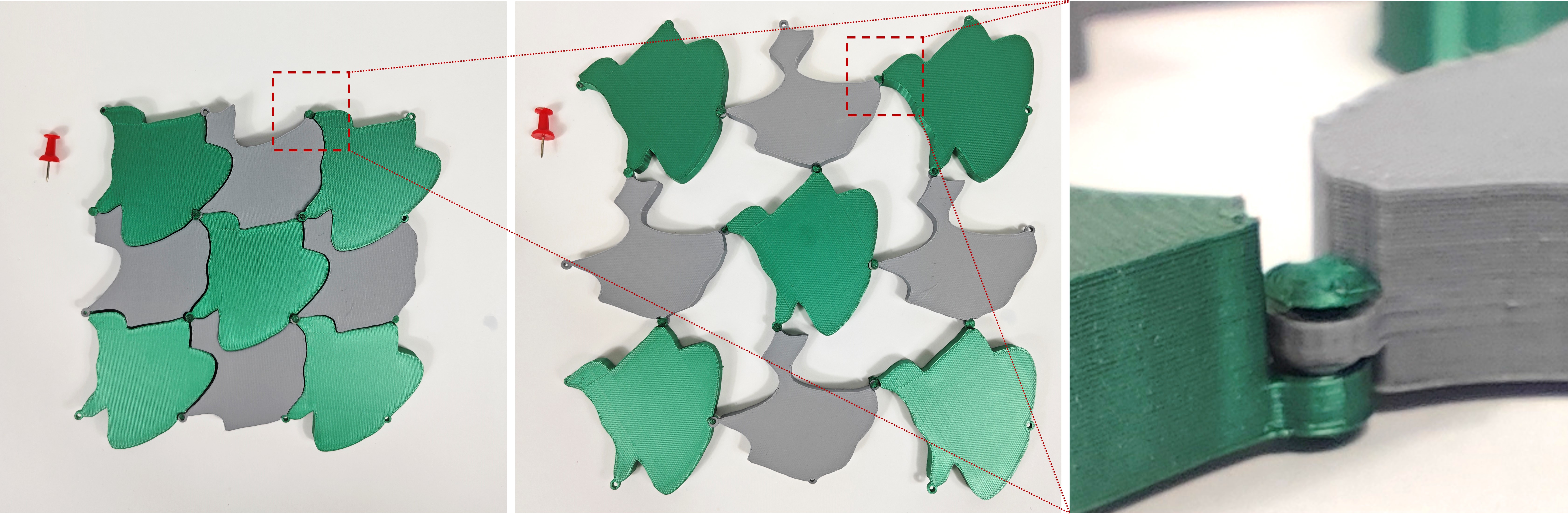
Joint design for large-scale manufacturing
Downloads
Acknowledgement
We thank all the anonymous reviewers for their valuable comments and constructive suggestions. This work is supported by the grant No.61972232 from National Nat- ural Science Foundation of China (NSFC) and the Key Research and Development Plan of Shandong Province of China (No.2020ZLYS01).
BibTex


